Posted in Algebra

How to derive the quadratic formula

Posted on by

As I wrote in my  earlier post about solving quadratic equations, introducing the quadratic formula in solving for the roots of a quadratic equation is not advisable because it does not promote conceptual understanding. All the students learn in using the formula is to substitute the values and evaluate the resulting numerical expression. I have seen test questions like “In 2x^2-4x+4=0, what is the value of a, b and c?”  Where is the mathematics in this item?

Not teaching the quadratic formula in solving for the roots of a quadratic equation does not mean that the quadratic formula will not be part of the algebra lesson. It would be a good exercise at the end of the unit to ask students to derive a formula for finding the roots of ax^2+bx+c=0 because you will be talking about vertex and discriminant (if you think they need to know what a discriminant is as this will just add to the terms they need to memorize) in later lessons. However I suggest that you ask the students ‘to solve for x’than ‘derive the formula’.

Problem

Solve the equation ax^2+bx+c=0.

Solution

 

 

 

 

 

 

Express the left hand side as product: x(x+ \frac {b}{a}) = \frac {-c}{a}.

Complete the square:

The rest as they say is pure algebra:

As you can see, deriving the quadratic formula is a beauty. Using it is not. Completing the square and factoring will do for students solving quadratic equations for the first time (ninth grade, for most countries). What is needed at this point is exposure to different problem solving context requiring representations of and solving quadratic equations.

Coming up in the next post is the meaning of this in graphs.

 

Author:

4 thoughts on “How to derive the quadratic formula”

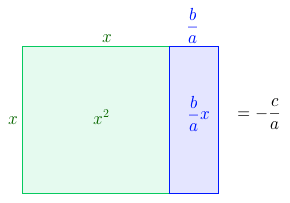
  1. Sorry but I don’t see the point of the first visual. The algebraic expression is x(x + (b/a)x): how is that related to the visual? (b/a)x represents the area of a rectangle with side lengths x and b/a, but what is the geometric meaning of x + (b/a)x ? What does it mean to add a linear measure with an area measure? The second visual I get, but still it does not help to facilitate the learning of how the formula is derived. The leap to b/2a still seems too artificial, or better, it does not come naturally. I mean, the visual explanation has the same flaw as the algebraic one of the formula.

    1. The x(x+b/a)x was a typo error. Thank you for pointing that out. I made the error when I typed using latex. I already corrected it to x(x+b/a). As for the leap from b/a to b/2a, I leave it to the reader to make the connection. If you want to use this in your class you can ask the students to divide the area b/a in two equal parts and put them at the two edges. You may then ask your students for the expression of the missing area to complete the figure into a square.

      You are right about the visuals and area model as having a ‘flaw’. In the first place, the variables can take negative values so technically you can’t use area model for it. I would not advise it. The visual is used here as an aid for making sense of the algebraic derivation of the formula.

  2. i like it but i wish it can be shown from the beginning up to the last part through algebra tiles.

Comments are closed.